 and
and
The grouping block determines the concurrent time-frequency region of the desired signal using constraints (i) and (iii) in Table 1, and then reconstructs the segregated instantaneous amplitude and phase using the inverse wavelet transform [Unoki and Akagi1999].
 and
and
![]() are the reconstructed f1(t) and f2(t).
are the reconstructed f1(t) and f2(t).
Constraint (i) is implemented by comparing the onset/offset (
![]() )
of Xk(t) with the onset/offset (
)
of Xk(t) with the onset/offset (
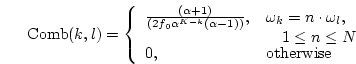 )
of
)
of
![]() corresponding to F0(t), where
corresponding to F0(t), where
![]() ms and
ms and
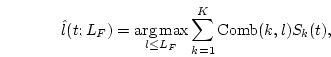 ms [Unoki and Akagi1999].
In this paper, onset
ms [Unoki and Akagi1999].
In this paper, onset
![]() and offset
and offset
![]() in Xk(t) are determined as follows.
in Xk(t) are determined as follows.
Constraint (iii) is implemented by determining the channel number corresponding to the integer multiples of F0(t).
The channel number ![]() of
of ![]() ,
in which the harmonic components exist in the output of the
,
in which the harmonic components exist in the output of the ![]() -th channel, is determined by
-th channel, is determined by